Definition of Mathematical Constants
‘A mathematical constant is a key number whose value is fixed by an unambiguous definition, often referred to by a symbol (e.g. an alphabet letter), or by mathematicians’ names to facilitate using it across multiple mathematical problems. Constants arise in many areas of mathematics, with constants such as e and π occurring in such diverse contexts as geometry, number theory, statistics and calculus.’
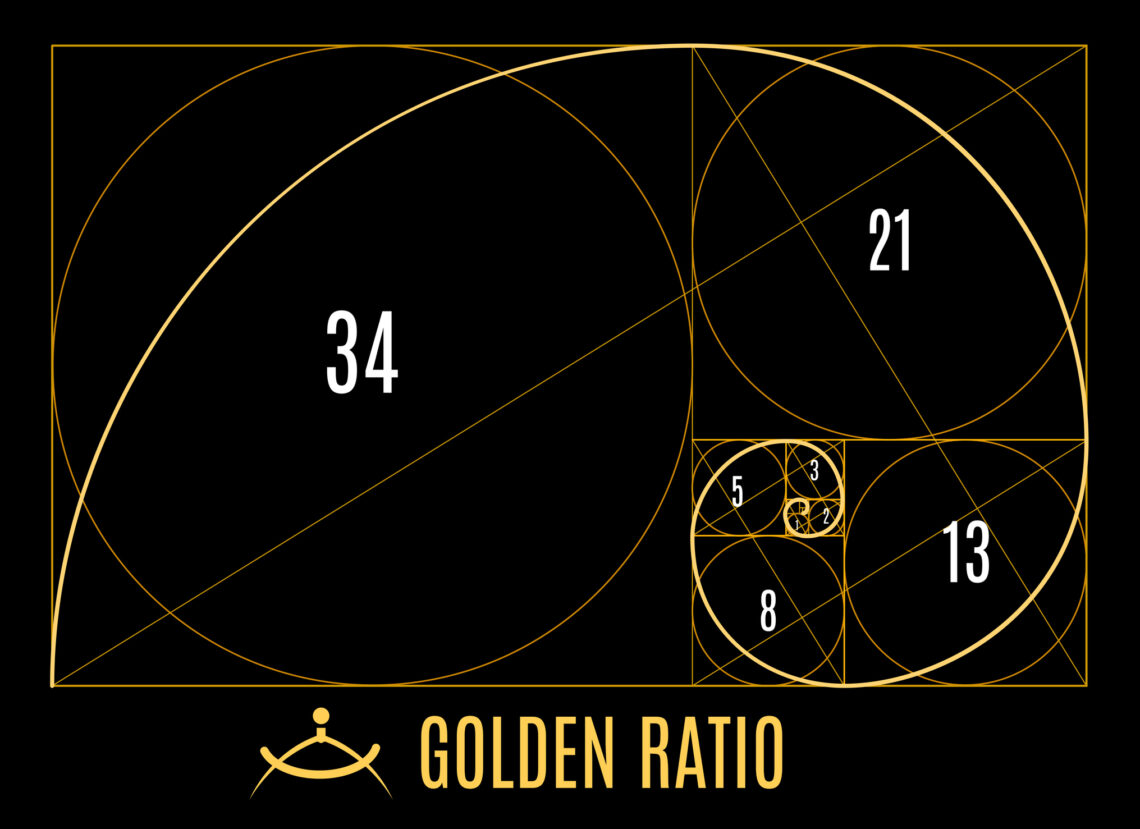
Below is a list of some of the Mathematical Constants that includes my favourite Golden Ratio, which Fibonacci made famous.
Mathematical Constants
Negative Numbers and Constants
The interesting thing is that all of them are positive except for two of them. The first of the non-positive is 0 and is neither positive nor negative. The second is the imaginary number √-1, which is not just a number but also a rotation of a certain magnitude. So, it’s an imaginary constant.
So are there no other negative Mathematical Constants? You could put a minus sign in front of any of them but that does not explain why they are all positive. They are positive because they emanate from the natural world where negative numbers do not exist. Negative numbers are not real.